What Are The Factors Of 63
Video What are the Elements of 63Factor 63 are integers that are equally divisible by unique numbers. There are all six factors of 63, i.e. 1, 3, 7, 9, 21 and 63. Therefore, the smallest problem is 1 and the best problem of 63 is 63, itself. The factors of the pair 63 are the numbers that, when multiplied by the pairs, will end up as a unique number. The factors in Pairs are (1, 63), (3, 21) and (7, 9). If we add up all the factors of 63, the possible sum will be: 1 + 3 + 7 + 9 + 21 + 63 = 104. using the division method and the prime factorization method. Also, see some examples involving the factors of 63. Read: What are the factors of 63
What are the factors of 63?
Contents
The factors of 63 are numbers that exactly divide 63 without discarding the remainder. In other words, the factors of the pair 63 are numbers that are multiplied in pairs resulting in the unique number 63. Since 63 is a composite quantity, 63 has more than two factors. Thus, the factors of 63 are 1, 3, 7, 9, 21 and 63.
Pair factor of 63
The factors of the 63 pair are numbers that are multiplied in pairs to give a product of value 63. Since the factors of 63 can be constructive or harmful, the factors of the 63 pair can be. constructive or harmful, however they cannot be in the form of fractions or decimals. Therefore, the constructive and damaging pair factors of 63 are given below.
Tips on how to discover elements out of 63?
63 is an odd number with six factors. Since the number of elements is much less, it is therefore simple to get these by using two easy strategies. They are:
- Division technique
- Key factor identification technique
Elements of 63 Used Parts Techniques
Using the easy division method, we can consider the factors of 63. Let us begin.
- Divide 63 by the smallest realizable divisor, i.e. 1. Thus, one of the coefficients of 63 is 1.
- Now verify with the next whole number, divisible by a full 63. 63/3 = 21. Therefore, 3 is a prime.
- Continue dividing by the whole number until we get 63/63 = 1. Because, we can’t take additional integers.
- Therefore, the factors that we obtain now are:
- 63/1 = 63
- 63/3 = 21
- 63/7 = 9
- 63/9 = 7
- 63/21 = 3
- 63/63 = 1
Therefore, the required coefficients of 63 are 1,3,7,9,21 and 63.
Use tricks
Another trick to find the factors of 63 using division method is given below:
- Divide 63 by 1 to get 63. (1 and 63 are factors)
- Divide 63 by 3 to get 21. (3 and 21 are factors)
- Now we all know 21 can also be a composite quantity.
- Divide 21 by 3, you get 7 (Once you add 7 and three are factors)
Thus, following the steps above, the numbers 1, 63, 21, 7 and three become a factor of 63. Since 3 is repeated twice, 3 x 3 = 9 can also be a factor of 63. Therefore, the full coefficients are 1, 3, 7, 9, 21 and 63.
Prime factor of 63
The number 63 is a composite quantity. Now allow us to explore the prime factors related to 63.
- Step one is to divide the number 63 by the smallest prime number, i.e. 2.
Read more: In which season do jackfruit and lisa get married63 ÷ 2 = 31.5; fraction cannot be an element. So go to next prime Divide 63 by 3.63 3 = 21
- Again divide 21 by 3 and keep reducing the output by 3 until you get 1 or a fraction.
21 ÷ 3 = 77 ÷ 3 = 2.33; cannot be an element. Now move on to the next prime number 7.7 7 = 1
- We got 1 at the end and it didn’t have any problems. Due to this fact, we cannot proceed with additional division method. So the prime factor of 63 is 3 × 3 × 7 or 32 × 7, positions 3 and seven are primes.
Video lesson on prime coefficients
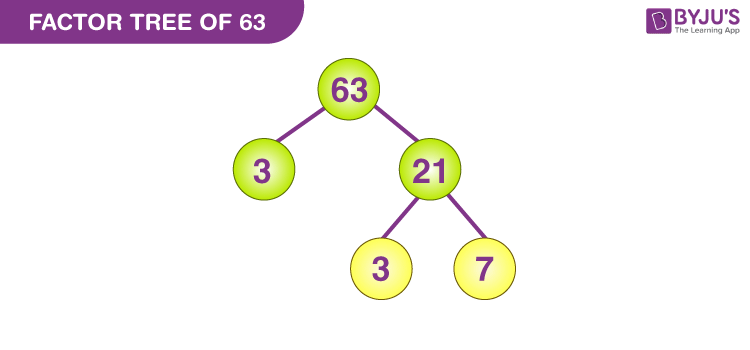
Problem tree number 63
By prime factorization, we have now seen, how we can divide the quantity 63 into prime factors. Therefore, the problem tree of such shape is proved in the lower definition.
Information about the elements of 63
- Factor 63 – 1, 3, 7, 9, 21 and 63
- Prime factors 63 – 3 × 3 × 7
- Prime numbers 63 – 3 and seven
- Match the coefficients 63 – (1, 63), (3, 21) and (7, 9)
- Sum of the factors of 63 – 104
Solved examples of the factors of 63
Case 1:Discover the frequent factors of 63 and 62.Resolution:The factors of 63 are 1, 3, 7, 9, 21 and 63 The factors of 62 are 1, 2, 31, 62. Read more: What sign is June 19 Thus, the common number of 63 and 62 is 1.Case 2:Discover the frequent factors of 63 and 64.Resolution:Factor 63 = 1, 3, 7, 9, 21 and 63 Factor 64 = 1, 2, 4, 8, 16, 32 and 64 Thus, the frequent factors of 63 and 64 are 1.Case 3:Discover the frequent factors of 63 and 61.Resolution: The factors of 63 are 1, 3, 7, 9, 21 and 63 The factors of 61 are 1 and 61 So the common numbers 63 and 61 are unique 1, because 61 is the principal quantity.
Practice questions on the elements of 63
Learn more about factors and primes right here with us in BYJU’S and beyond, download BYJU’S – Learning App for further expertise and get video content material to test and perceive ideas ideas of Math problems. Read more: What is mac medical coding.
Last, Wallx.net sent you details about the topic “What Are The Factors Of 63❤️️”.Hope with useful information that the article “What Are The Factors Of 63” It will help readers to be more interested in “What Are The Factors Of 63 [ ❤️️❤️️ ]”.
Posts “What Are The Factors Of 63” posted by on 2022-05-20 12:36:02. Thank you for reading the article at wallx.net